 Image via Wikipedia
Image via WikipediaIn our last posting, we discussed the notion of asymptotes, and I posed two problems for your consideration. One involved the eventual (but unreachable) sum of a convergent series of numbers, and the other involving a ever-more troubling fraction. You can quickly refresh your memory by clicking on http://braintenance.blogspot.com/2011/09/asymptotes-closer-but-never.html, and by then hitting your browser's "BACK" button.
The answers are unsatisfying, but they were promised:
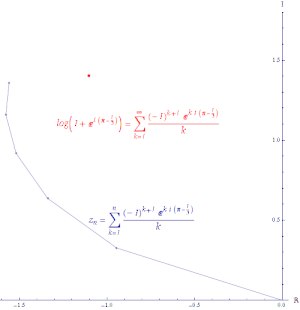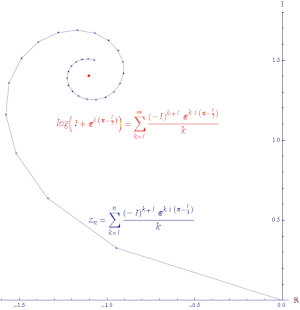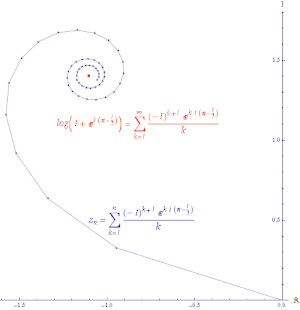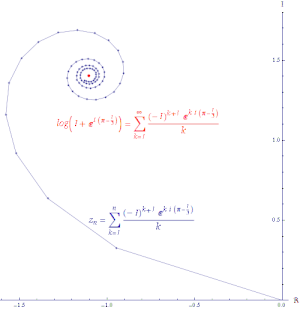
1) In adding the sum of the series 1 + 1/2 + 1/4 + 1/8....and so forth, the sum will eventually approach, but never quite reach a limit of 2.
2) In dividing (n-1)/n, as n increases, the value of the expression approaches, but never reaches 1.
There are examples of this type of complex conundrum in nature, in such things as trying to solve 22/7 (which is a never-ending decimal), and in determining the halflives of certain radioactive materials (isotopes), where one half of the material loses its radioactive potency over a certain period of time, but the residual amount keeps getting halved and never quite disappears.
I solemnly promise to offer you something more nifty in my next article. The idea is to tax your brain until it has to expand its capacity in order to solve increasingly complex problems.
Sadly (and speaking about interesting wordplay and punnery), taxing our brains , while making us brighter, still won't make up the federal deficit... Did I hear somebody groaning?
Douglas E Castle


No comments:
Post a Comment